MAKE A MEME
View Large Image
| View Original: | Newton-Fractal 2z3-2z+2.png (1023x768) | |||
| Download: | Original | Medium | Small | Thumb |
| Courtesy of: | commons.wikimedia.org | More Like This | ||
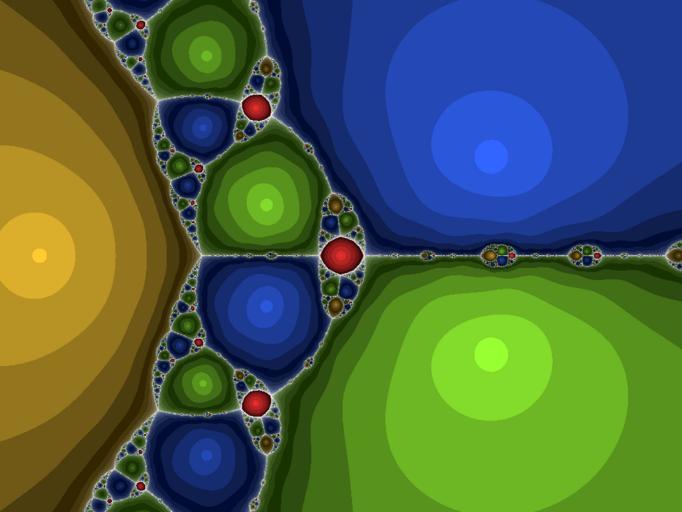
| Keywords: Newton-Fractal 2z3-2z+2.png Newton fractal for the polynomial <math>f z\mapsto z 3-2z+2</math> in the range -2 2 × -1 5 1 5<math>i</math> Numbers in the orange green and blue basins converge to zeros of <math>f</math> numbers in the red basins are attracted by the attractive cycle <math>\ 0 1\ </math> i e they do not converge to a zero of <math>f</math> The white structure is the Newton fractal for <math>f</math> i e it is the Julia set <math>J</math> for the meromorphic function <math>g z\mapsto z-\frac f z f' z 2\frac z 3-1 3z 2-2 </math> Zeros of <math>f</math> are attractive fixpoints of <math>g</math> Moreover 1 and 0 are fixpoints of <math>g\circ g</math> but not of <math>g</math> The set <math>J</math> has the following properties its interior is empty it is closed <math>\ J \ \mathbb R </math> <math>J</math> is connected <math>J \partial B_\mathrm red \partial B_\mathrm orange \partial B_\mathrm blue \partial B_\mathrm green </math> where <math>\partial B</math> denotes the border of a basin This means that every point in <math>J</math> is a 4-corner point own 2008-03-20 Georg-Johann Lay thumb Closeup using the same color map Newton fractals | ||||